Chapter 1: Introduction to Snowflakes

Snowflakes are not only stunning but also intricate formations that arise when water vapor condenses and freezes in the atmosphere. These delicate structures exhibit a hexagonal symmetry, a result of the molecular interactions of water. The mathematical principles that govern snowflakes include geometry, symmetry, and group theory.

Geometry plays a crucial role in understanding the formation and development of snowflakes by detailing the shapes and arrangements of water molecules and crystals. For instance, water molecules adopt a tetrahedral shape, which enables them to bond and form hexagonal rings. These rings subsequently arrange into a hexagonal lattice, the fundamental unit of a snowflake. As the snowflake descends, it gathers additional water molecules, resulting in the formation of branches and intricate patterns that are influenced by the surrounding temperature and humidity.

Chapter 2: The Role of Symmetry

Symmetry is essential for understanding the appearance and behavior of snowflakes, as it describes the invariance and patterns inherent in their crystal structures. A snowflake possesses six-fold rotational symmetry, meaning it appears identical when rotated by 60 degrees. Additionally, it has three reflection symmetries, allowing it to maintain its appearance when flipped across three distinct axes. These symmetries arise from the hexagonal configuration of the water molecules and the resulting crystals.

Group theory, a mathematical field focused on the properties of groups—sets of elements combined under specific rules—further assists in understanding the classification and variety of snowflakes. For example, snowflakes belong to a group known as D6, which consists of 12 elements: six rotations and six reflections. Each element within this group signifies a transformation that maintains the snowflake's symmetry.

The D6 group is subdivided into six subgroups, representing various types of snowflakes with varying degrees of symmetry: D3, D2, C6, C3, C2, and C1. Here, D indicates dihedral symmetry (two types) while C stands for cyclic symmetry (one type), with the number denoting the group order (the count of elements).

Chapter 3: Exploring Fractal Geometry

Snowflakes also demonstrate fractal characteristics, featuring self-similar structures at multiple scales. The concept of fractals can be mathematically represented through recursive algorithms. A notable example is the Koch snowflake, a mathematical representation that mirrors the repeating, self-similar patterns found in actual snowflakes.

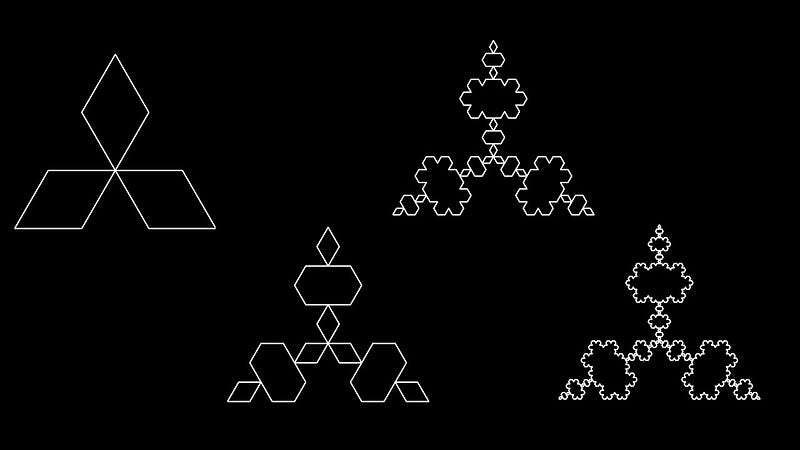
The mathematics of fractal snowflakes is brilliantly elaborated by Dr. Trefor Bazett.

In this video titled "Fractal Snowflakes, Symmetries, and Beautiful Math Decorations," Dr. Bazett delves into the intricate mathematical concepts that shape the world of snowflakes.

The second video, "The Mathematics Behind Pineapples, Snowflakes and Beyond," explores the mathematical principles that connect various natural phenomena, including snowflakes.

Thank you for taking the time to read this exploration of snowflakes. If you found this information engaging, please feel free to show your appreciation! For those interested in supporting my work, consider buying me a coffee. Stay tuned for more captivating stories!